CHSE Odisha Class 12 Math Solutions Chapter 2 Inverse Trigonometric Functions Exercise 2

CHSE Odisha Class 12 Math Solutions Chapter 2 Inverse Trigonometric Functions Exercise 2

Question 1.
Fill in the blanks choosing correct answer from the brackets:
(i) If A = tan^-1 x, then the value of sin 2A = ________. (\(\frac{2 x}{1-x^2}\), \(\frac{2 x}{\sqrt{1-x^2}}\), \(\frac{2 x}{1+x^2}\))
Solution:
\(\frac{2 x}{1+x^2}\)

(ii) If the value of sin^-1 x = \(\frac{\pi}{5}\) for some x ∈ (-1, 1) then the value of cos^-1 x is ________. (\(\frac{3 \pi}{10}\), \(\frac{5 \pi}{10}\),\(\frac{3 \pi}{10}\))
Solution:
\(\frac{3 \pi}{10}\)

(iii) The value of tan^-1 x (2cos\(\frac{\pi}{3}\)) is ________. (1, \(\frac{\pi}{4}\), \(\frac{\pi}{3}\))
Solution:
\(\frac{\pi}{4}\)

(iv) If x + y = 4, xy = 1, then tan^-1 x + tan^-1 y = ________. (\(\frac{3 \pi}{4}\), \(\frac{\pi}{4}\), \(\frac{\pi}{3}\))
Solution:
\(\frac{\pi}{2}\)

(v) The value of cot^-1 2 + tan^-1 \(\frac{1}{3}\) = ________. (\(\frac{\pi}{4}\), 1, \(\frac{\pi}{2}\))
Solution:
\(\frac{\pi}{4}\)

(vi) The principal value of sin^-1 (sin \(\frac{2 \pi}{3}\)) is ________. (\(\frac{2 \pi}{3}\), \(\frac{\pi}{3}\), \(\frac{4 \pi}{3}\))
Solution:
\(\frac{\pi}{3}\)

(vii) If sin^-1 \(\frac{x}{5}\) + cosec^-1 \(\frac{5}{4}\) = \(\frac{\pi}{2}\), then the value of x = ________. (2, 3, 4)
Solution:
x = 3

(viii) The value of sin (tan^-1 x + tan^-1 \(\frac{1}{x}\)), x > 0 = ________. (0, 1, 1/2)
Solution:
1

(ix) cot^-1 \(\left[\frac{\sqrt{1-\sin x}+\sqrt{1+\sin x}}{\sqrt{1-\sin x}-\sqrt{1+\sin x}}\right]\) = ________. (2π – \(\frac{x}{2}\), \(\frac{x}{2}\), π – \(\frac{x}{2}\))
Solution:
π – \(\frac{x}{2}\)

(x) 2sin^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{24}{25}\) = ________. (π, -π, 0)
Solution:
π

(xi) if Θ = cos^-1 x + sin^-1 x – tan^-1 x, x ≥ 0, then the smallest interval in which Θ lies is ________. [(\(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\)), [0, \(\frac{\pi}{2}\)), (0, \(\frac{\pi}{2}\)])
Solution:
(0, \(\frac{\pi}{2}\)]

(xii) sec² (tan^-1 2) + cosec² (cot^-1 3) = ________. (16, 14, 15)
Solution:
15

Question 2.
Write whether the following statements are true or false.
(i) sin^-1 \(\frac{1}{x}\) cosec^-1 x = 1
Solution:
False

(ii) cos^-1 \(\frac{4}{5}\) + tan^-1 \(\frac{2}{3}\) = tan^-1 \(\frac{17}{6}\)
Solution:
True

(iii) tan^-1 \(\frac{4}{3}\) + cot^-1 (\(\frac{-3}{4}\)) = π
Solution:
True

(iv) sec^-1 \(\frac{1}{2}\) + cosec^-1 \(\frac{1}{2}\) = \(\frac{\pi}{2}\)
Solution:
False

(v) sec^-1 (-\(\frac{7}{5}\)) = π – cos^-1 \(\frac{5}{7}\)
Solution:
True

(vi) tan^-1 (tan 3) = 3
Solution:
False

(vii) The principal value of tan^-1 (tan \(\frac{3 \pi}{4}\)) is \(\frac{3 \pi}{4}\)
Solution:
False

(viii) cot^-1 (-√3) is in the second quadrant.
Solution:
True

(ix) 3 tan^-1 3 = tan^-1 \(\frac{9}{13}\)
Solution:
False

(x) tan^-1 2 + tan^-1 3 = – \(\frac{\pi}{4}\)
Solution:
False

(xi) 2 sin^-1 \(\frac{4}{5}\) = sin^-1 \(\frac{24}{25}\)
Solution:
False

(xii) The equation tan^-1 (cotx) = 2x has exactly two real solutions.
Solution:
True

Question 3.
Express the value of the folowing in simplest form.
(i) sin (2 sin^-1 0.6)
Solution:
sin (2 sin^-1 0.6)

(ii) tan (\(\frac{\pi}{4}\) + 2 cot^-1 3)
Solution

:

(iii) cos (2 sin^-1 x)

Solution:

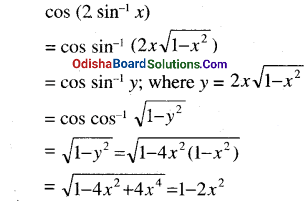
(iv) tan (cos^-1 x)
Solution:

(v) tan^-1 (\(\frac{x}{y}\)) – tan^-1 \(\frac{x-y}{x+y}\)
Solution:

(vi) cosec (cos^-1 \(\frac{3}{5}\) + cos^-1 \(\frac{4}{5}\))
Solution:

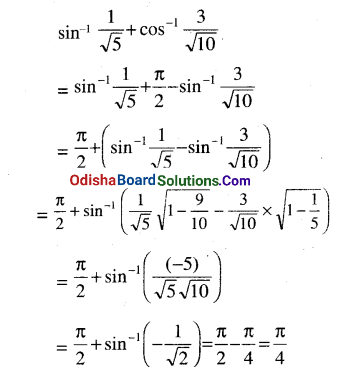
(vii) sin^-1 \(\frac{1}{\sqrt{5}}\) + cos^-1 \(\frac{3}{\sqrt{10}}\)
Solution:

(viii) sin cos^-1 tan sec √2
Solution:
sin cos^-1 tan sec √2
= sin cos^-1 tan sec \(\frac{\pi}{4}\)
= sin cos^-1 1 = sin 0 = 0

(ix) sin (2 tan^-1 \(\sqrt{\frac{1-x}{1+x}}\))
Solution:

(x) tan \(\left\{\frac{1}{2} \sin ^{-1} \frac{2 x}{1+x^2}+\frac{1}{2} \cos ^{-1} \frac{1-y^2}{1+y^2}\right\}\)
Solution:

(xi) sin cot^-1 cos tan^-1 x.
Solution:

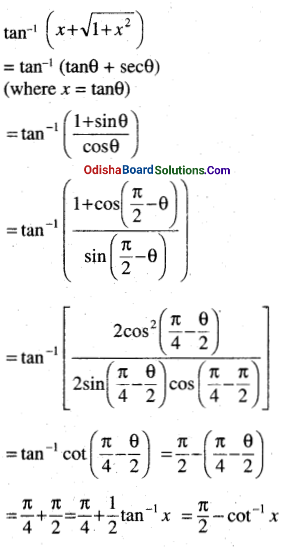
(xii) tan^-1 \(\left(x+\sqrt{1+x^2}\right)\)
Solution:

Question 4.
Prove the following statements:
(i) sin^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{8}{17}\) = cos^-1 \(\frac{36}{85}\)
Solution:

L.H.S = sin^-1 \(\frac{3}{5}\) + sin^-1 \(\frac{8}{17}\)

(ii) sin^-1 \(\frac{3}{5}\) + cos^-1 \(\frac{12}{13}\) = cos^-1 \(\frac{33}{65}\)
Solution:

L.H.S = sin^-1 \(\frac{3}{5}\) + cos^-1 \(\frac{12}{13}\)

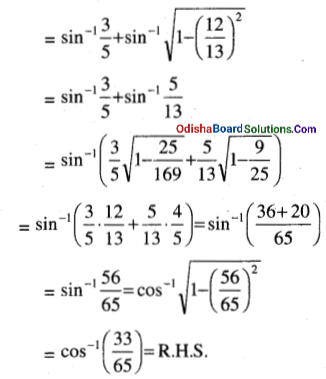
Or

Let α = sin^-1 (³/₅) and β = cos^-1 (¹²/₁₃).

Find cos α and sin β:
cos α = ⁴/₅
sin β = ⁵/₁₃

Calculate cos (α + β) using:
cos (α + β) = cos α cos β – sin α sin β
cos (α + β) = ⁴/₅ × ¹²/₁₃ – ³/₅ × ⁵/₁₃ = ⁴⁸/₆₅ – ¹⁵/₆₅ = ³³/₆₅

Thus:
sin^-1 (³/₅) + cos^-1 (¹²/₁₃) = cos^-1 (³³/₆₅)

(iii) tan^-1 \(\frac{1}{7}\) + tan^-1 \(\frac{1}{13}\) = tan^-1 \(\frac{2}{9}\)
Solution:
L.H.S = tan^-1 \(\frac{1}{7}\) + tan^-1 \(\frac{1}{13}\)

(iv) tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{5}\) + tan^-1 \(\frac{1}{8}\) = \(\frac{\pi}{4}\)
Solution:

L.H.S = tan^-1 \(\frac{1}{2}\) + tan^-1 \(\frac{1}{5}\) + tan^-1 \(\frac{1}{8}\)

(v) tan ( 2tan^-1 \(\frac{1}{5}\) – \(\frac{\pi}{4}\) ) + \(\frac{7}{17}\) = 0
Solution:

tan ( 2tan^-1 \(\frac{1}{5}\) – \(\frac{\pi}{4}\) ) + \(\frac{7}{17}\)

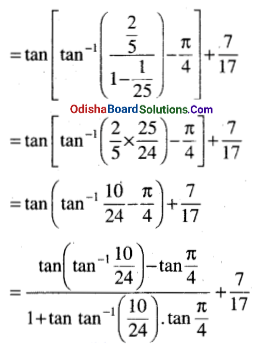
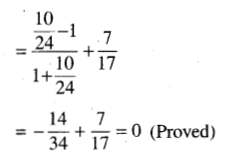
Question 5.
Prove the following statements:
(i) cot^-1 9 + cosec^-1 \(\frac{\sqrt{41}}{4}\) = \(\frac{\pi}{4}\)
Solution: L.H.S= cot^-1 9 + cosec^-1 \(\frac{\sqrt{41}}{4}\) 

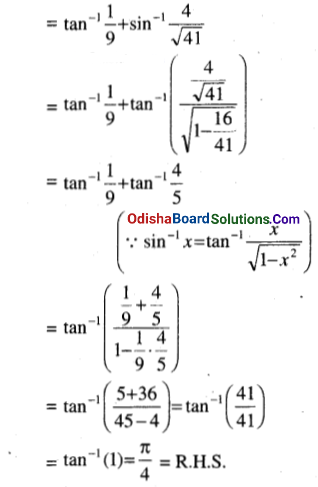
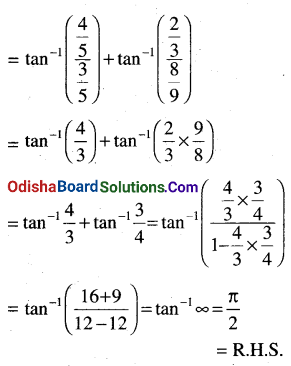
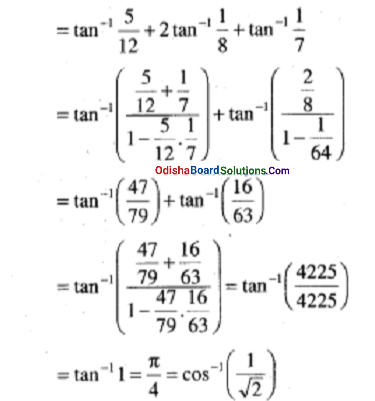
(ii) sin^-1 \(\frac{4}{5}\) + 2 tan^-1 \(\frac{1}{3}\) = \(\frac{\pi}{2}\)
Solution:

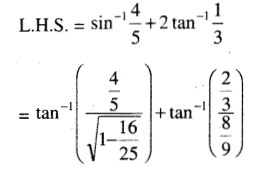
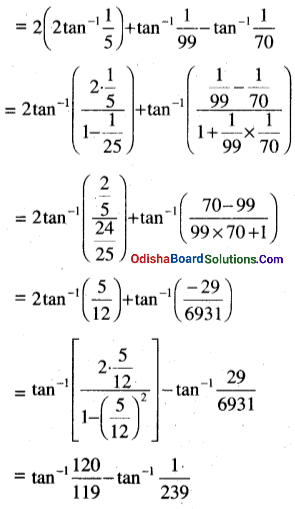
(iii) 4 tan^-1 \(\frac{1}{5}\) – tan^-1 \(\frac{1}{70}\) + tan^-1 \(\frac{1}{99}\) = \(\frac{\pi}{4}\)
Solution:

L.H.S

= 4 tan^-1 \(\frac{1}{5}\) – tan^-1 \(\frac{1}{70}\) + tan^-1 \(\frac{1}{99}\)

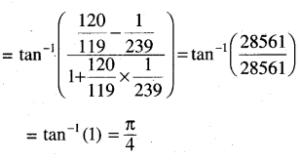
(iv) 2 tan^-1 \(\frac{1}{5}\) + sec^-1 \(\frac{5 \sqrt{2}}{7}\) + 2 tan^-1 \(\frac{1}{8}\) = \(\frac{\pi}{4}\)
Solution:

2 tan^-1 \(\frac{1}{5}\) + sec^-1 \(\frac{5 \sqrt{2}}{7}\) + 2 tan^-1 \(\frac{1}{8}\)

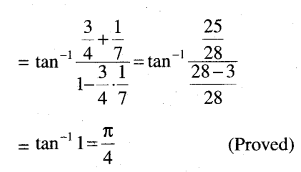
(v) cos^-1 \(\frac{12}{13}\) + 2 cos^-1 \(\sqrt{\frac{64}{65}}\) + cos^-1 \(\sqrt{\frac{49}{50}}\) = cos^-1 \(\frac{1}{\sqrt{2}}\)
Solution:

cos^-1 \(\frac{12}{13}\) + 2 cos^-1 \(\sqrt{\frac{64}{65}}\) + cos^-1 \(\sqrt{\frac{49}{50}}\)

(vi) tan² cos^-1 \(\frac{1}{\sqrt{3}}\) + cot² sin^-1 \(\frac{1}{\sqrt{5}}\) = 6
Solution:
tan² cos^-1 \(\frac{1}{\sqrt{3}}\) + cot² sin^-1 \(\frac{1}{\sqrt{5}}\)
= tan² tan^-1 √2 + cot² cot^-1 (2)
= 2 + 4 = 6

(vii) cos tan^-1 cot sin^-1 x = x.
Solution.

Question 6.
Prove the following statements:
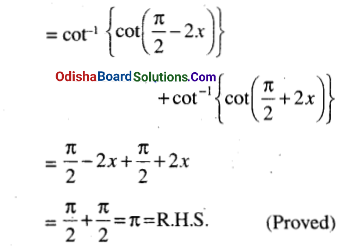
(i) cot^-1 (tan 2x) + cot^-1 (- tan 2x) = π
Solution:
L.H.S = cot^-1 (tan 2x) + cot^-1 (- tan 2x)

(ii) tan^-1 x + cot^-1 (x + 1) = tan^-1 (x² + x + 1)
Solution:

L.H.S = tan^-1 x + cot^-1 (x + 1)

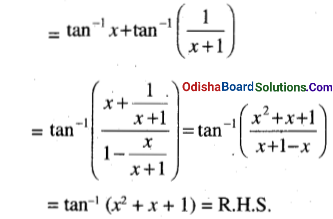
(iii) tan^-1 (\(\frac{a-b}{1+a b}\)) + tan^-1 (\(\frac{b-c}{1+b c}\)) = tan^-1 a – tan^-1 c.
Solution:
tan^-1 (\(\frac{a-b}{1+a b}\)) + tan^-1 (\(\frac{b-c}{1+b c}\))
= tan^-1 a – tan^-1 b + tan^-1 b – tan^-1 c
= tan^-1 a – tan^-1 c.

(iv) cot^-1 \(\frac{p q+1}{p-q}\) + cot^-1 \(\frac{q r+1}{q-r}\) + cot^-1 \(\frac{r p+1}{r-p}\) = 0
Solution:

L.H.S = cot^-1 \(\frac{p q+1}{p-q}\) + cot^-1 \(\frac{q r+1}{q-r}\) + cot^-1 \(\frac{r p+1}{r-p}\)

(v)

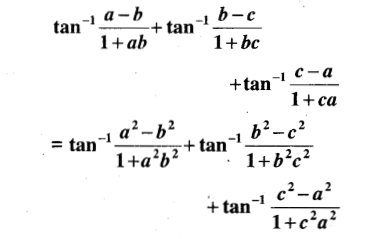
Solution:

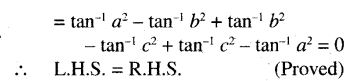
Question 7.
Prove the following statements:
(i) tan^-1 \(\frac{2 a-b}{b \sqrt{3}}\) + tan^-1 \(\frac{2 b-a}{a \sqrt{3}}\) = \(\frac{\pi}{3}\)
Solution:

L.H.S = tan^-1 \(\frac{2 a-b}{b \sqrt{3}}\) + tan^-1 \(\frac{2 b-a}{a \sqrt{3}}\)

(ii) tan^-1 \(\frac{1}{x+y}\) + tan^-1 \(\frac{y}{x^2+x y+1}\) = tan^-1 \(\frac{1}{x}\)
Solution:

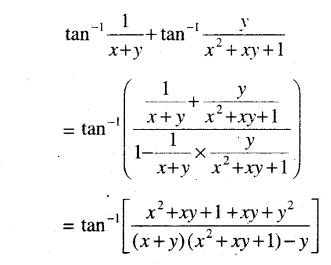
(iii) sin^-1 \(\sqrt{\frac{x-q}{p-q}}\) = cos^-1 \(\sqrt{\frac{p-x}{p-q}}\) = cot^-1 \(\sqrt{\frac{p-x}{x-q}}\)
Solution:

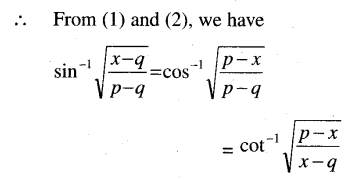
(iv) sin² (sin^-1 x + sin^-1 y + sin^-1 z) = cos² (cos^-1 x + cos^-1 y + cos^-1 z)
Solution:

L.H.S = sin² (sin^-1 x + sin^-1 y + sin^-1 z)

(v) tan (tan^-1 x + tan^-1 y + tan^-1 z) = cot (cot^-1 x + cot^-1 y + cot^-1 z)
Solution:

L.H.S = tan (tan^-1 x + tan^-1 y + tan^-1 z)

Question 8.
(i) If sin^-1 x + sin^-1 y + sin^-1 z = π, show that x\(\sqrt{1-x^2}\) + x\(\sqrt{1-y^2}\) + x\(\sqrt{1-z^2}\) = 2xyz
Solution:
Let sin^-1 x = α, sin^-1 y = β, sin^-1 z = γ
∴ α + β + γ = π
∴ x = sin α, y = sin β, z = sin γ
or, α + β = π – γ
or, sin(α + β) = sin(π – γ) = sin γ
and cos(α + β) = cos(π – γ) = – cos γ

L.H.S = x\(\sqrt{1-x^2}\) + x\(\sqrt{1-y^2}\) + x\(\sqrt{1-z^2}\)

(ii) tan^-1 x + tan^-1 y + tan^-1 z = π show that x + y + z = xyz.
Solution:

Given , tan^-1 x + tan^-1 y + tan^-1 z = π

(iii) tan^-1 x + tan^-1 y + tan^-1 z = \(\frac{\pi}{2}\). Show that xy + yz + zx = 1
Solution:

Given ,tan^-1 x + tan^-1 y + tan^-1 z = \(\frac{\pi}{2}\)

or, 1 – xy – yz – zx = 0
⇒ xy + yz + zx = 1

(iv) If r² = x² +y² + z², Prove that tan^-1 \(\frac{y z}{x r}\) + tan^-1 \(\frac{z x}{y r}\) + tan^-1 \(\frac{x y}{z r}\) = \(\frac{\pi}{2}\)
Solution:

L.H.S = tan^-1 \(\frac{y z}{x r}\) + tan^-1 \(\frac{z x}{y r}\) + tan^-1 \(\frac{x y}{z r}\)

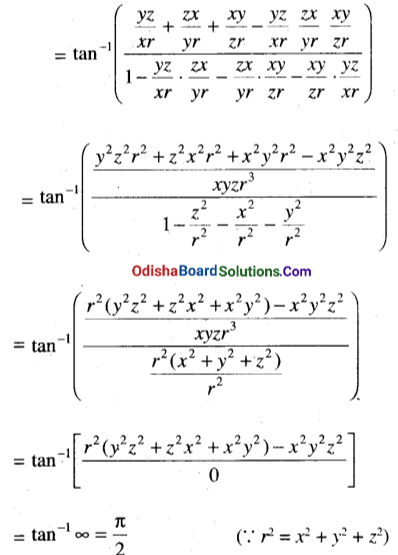
(v) In a triangle ABC if m∠A = 90°, prove that tan^-1 \(\frac{b}{a+c}\) + tan^-1 \(\frac{c}{a+b}\) = \(\frac{\pi}{4}\). where a, b, and c are sides of the triangle.
Solution:
L.H.S. tan^-1 \(\frac{b}{a+c}\) + tan^-1 \(\frac{c}{a+b}\)

Question 9.
Solve
(i) cos (2 sin^-1 x) = 1/9
Solution:

(ii) sin^-1 x + sin^-1 (1 – x) = \(\frac{\pi}{2}\)
Solution:
sin^-1 x + sin^-1 (1 – x) = \(\frac{\pi}{2}\)
or, sin^-1 (1 – x) = \(\frac{\pi}{2}\) – sin^-1 x = cos^-1 x
or, sin^-1 (1 – x) = sin^-1 \(\sqrt{1-x^2}\)
or, 1 – x = \(\sqrt{1-x^2}\)
or, 1 + x² – 2x = 1 – x²
or, 2x² – 2x = 0
or, 2x (x – 1) = 0
∴ x = 0 or, 1

(iii) sin^-1 (1 – x) – 2 sin^-1 x = \(\frac{\pi}{2}\)
Solution:
sin^-1 (1 – x) – 2 sin^-1 x = \(\frac{\pi}{2}\)
⇒ – 2 sin^-1 x = \(\frac{\pi}{2}\) – sin^-1 (1 – x)
⇒ cos^-1 (1 – x)
⇒ cos (– 2 sin^-1 x) = 1 – x ….. (1)
Let sin^-1 Θ ⇒ sin Θ
Now cos (– 2 sin^-1 x) = cos (-2Θ)
= cos 2Θ = 1 – 2 sin² Θ = 1 – 2x²
Using in (1) we get
1 – 2x² = 1 – x
⇒ 2x² – x = 0 ⇒ x (2x – 1) = 0
⇒ x = 0, ½, But x = ½ does not
Satisfy the given equation, Thus x = 0.

(iv) cos^-1 x + sin^-1 \(\frac{x}{2}\) = \(\frac{\pi}{6}\)
Solution:

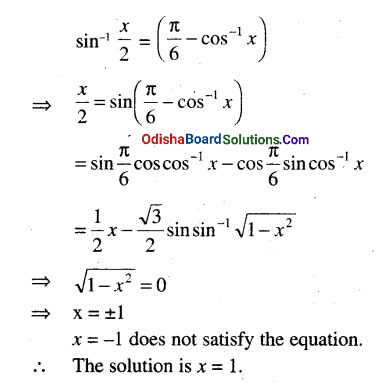
(v) tan^-1 \(\frac{x-1}{x-2}\) + tan^-1 \(\frac{x+1}{x+2}\) = \(\frac{\pi}{4}\)
Solution:

tan^-1 \(\frac{x-1}{x-2}\) + tan^-1 \(\frac{x+1}{x+2}\) = \(\frac{\pi}{4}\)

(vi) tan^-1 \(\frac{1}{2 x+1}\) + tan^-1 \(\frac{1}{4 x+1}\) = tan^-1 \(\frac{2}{x^2}\)
Solution:

tan^-1 \(\frac{1}{2 x+1}\) + tan^-1 \(\frac{1}{4 x+1}\) = tan^-1 \(\frac{2}{x^2}\)

(vii) 3 sin^-1 \(\frac{2 x}{1+x^2}\) – 4 cos^-1 \(\frac{1-x^2}{1+x^2}\) + 2 tan^-1 \(\frac{2 x}{1-x^2}\) = \(\frac{\pi}{3}\)
Solution:

3 sin^-1 \(\frac{2 x}{1+x^2}\) – 4 cos^-1 \(\frac{1-x^2}{1+x^2}\) + 2 tan^-1 \(\frac{2 x}{1-x^2}\) = \(\frac{\pi}{3}\)

(viii) cot^-1 \(\frac{1}{x-1}\) + cot^-1 \(\frac{1}{x}\) + cot^-1 \(\frac{1}{x+1}\) = cot^-1 \(\frac{1}{3x}\)
Solution:

cot^-1 \(\frac{1}{x-1}\) + cot^-1 \(\frac{1}{x}\) + cot^-1 \(\frac{1}{x+1}\) = cot^-1 \(\frac{1}{3x}\)

(ix) cot^-1 \(\frac{1-x^2}{2 x}\) = cosec^-1 \(\frac{1+a^2}{2 a}\) – sec^-1 \(\frac{1+b^2}{1-b^2}\)
Solution:

cot^-1 \(\frac{1-x^2}{2 x}\) = cosec^-1 \(\frac{1+a^2}{2 a}\) – sec^-1 \(\frac{1+b^2}{1-b^2}\)

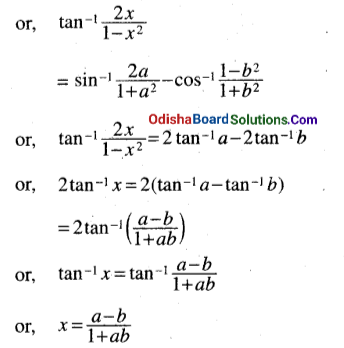
(x) sin^-1 \(\left(\frac{2 a}{1+a^2}\right)\) + sin^-1 \(\left(\frac{2 b}{1+b^2}\right)\) = 2 tan^-1 x
Solution:

sin^-1 \(\left(\frac{2 a}{1+a^2}\right)\) + sin^-1 \(\left(\frac{2 b}{1+b^2}\right)\) = 2 tan^-1 x

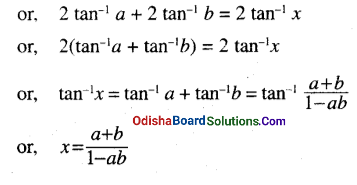
(xi) sin^-1 y – cos^-1 x = cos^-1 \(\frac{\sqrt{3}}{2}\)
Solution:

sin^-1 y – cos^-1 x = cos^-1 \(\frac{\sqrt{3}}{2}\)

(xii) sin^-1 2x + sin^-1 x = \(\frac{\pi}{3}\)
Solution:

sin^-1 2x + sin^-1 x = \(\frac{\pi}{3}\)

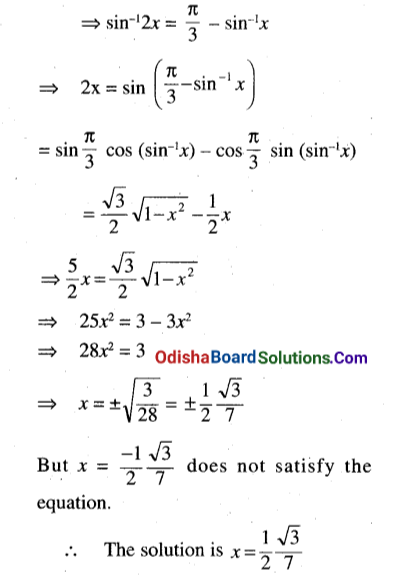
Question 10.
Rectify the error ifany in the following:
sin^-1 \(\frac{4}{5}\) + sin^-1 \(\frac{12}{13}\) + sin^-1 \(\frac{33}{65}\)
Solution:

Question 11.
Prove that:
(i) cos^-1 \(\left(\frac{b+a \cos x}{a+b \cos x}\right)\) = 2 tan^-1 \(\left(\sqrt{\frac{a-b}{a+b}} \tan \frac{x}{2}\right)\)
Solution:

(ii) tan \(\left(\frac{\pi}{4}+\frac{1}{2} \cos ^{-1} \frac{a}{b}\right)\) + tan \(\left(\frac{\pi}{4}-\frac{1}{2} \cos ^{-1} \frac{a}{b}\right)\)
Solution

(iii) tan^-1 \(\sqrt{\frac{x r}{y z}}\) + tan^-1 \(\sqrt{\frac{y r}{y x}}\) + tan^-1 \(\sqrt{\frac{z r}{x y}}\) = π where r = x + y +z.
Solution:

Question 12.
(i) If cos^-1 (\(\frac{x}{a}\)) + cos^-1 (\(\frac{y}{b}\)) = Θ, prove that \(\frac{x^2}{a^2}\) – \(\frac{2 x}{a b}\) cos Θ + \(\frac{y^2}{b^2}\) = sin² Θ.
Solution:

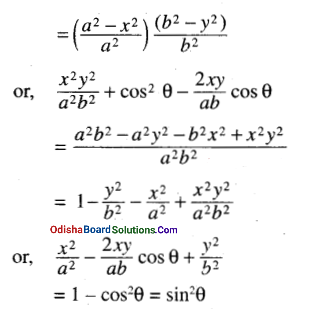
(ii) If cos^-1 (\(\frac{x}{y}\)) + cos^-1 (\(\frac{y}{3}\)) = Θ, prove that 9x² – 12xy cos Θ + 4y² = 36 sin² Θ.
Solution:

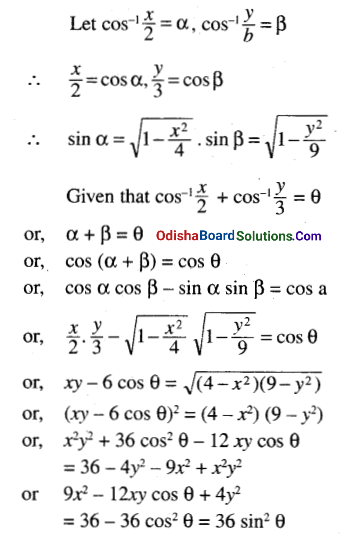
(iii) If sin^-1 (\(\frac{x}{a}\)) + sin^-1 (\(\frac{y}{b}\)) = sin^-1 (\(\frac{c^2}{a b}\)) prove that b²x² + 2xy \(\sqrt{a^2 b^2-c^4}\) a²y² = c²
Solution:

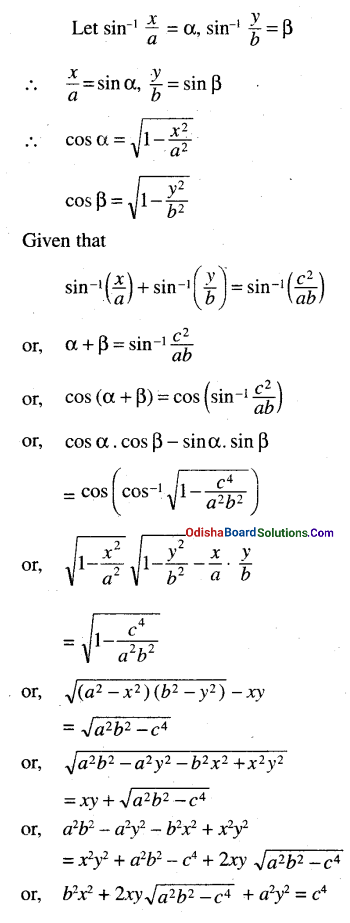
(iv) If sin^-1 (\(\frac{x}{a}\)) + sin^-1 (\(\frac{y}{b}\)) = α prove that \(\frac{x^2}{a^2}\) + \(\frac{2 x y}{a b}\) cos α + \(\frac{y^2}{b^2}\) = sin² α
Solution:

(v) If sin^-1 x + sin^-1 y + sin^-1 z = π prove that x² + y² + z² + 4x²y²z² = 2 ( x²y² + y²z² + z²x² )
Solution:

Question 13.
Solve the following equations:
(i) tan^-1 \(\frac{x-1}{x+1}\) + tan^-1 \(\frac{2 x-1}{2 x+1}\) = tan^-1 \(\frac{23}{36}\)
Solution:

(ii) tan^-1 \(\frac{1}{3}\) + tan^-1 \(\frac{1}{5}\) + tan^-1 \(\frac{1}{7}\) + tan^-1 x = \(\frac{\pi}{4}\)
Solution:

(iii) cos^-1 \(\left(x+\frac{1}{2}\right)\) + cos^-1 x+ cos^-1 \(\left(x-\frac{1}{2}\right)\) = \(\frac{3 \pi}{2}\)
Solution:

(iv) 3tan^-1 \(\frac{1}{2+\sqrt{3}}\) – tan^-1 \(\frac{1}{x}\) = tan^-1 \(\frac{1}{3}\)
Solution: